| Problem statement Solution video |
DISCUSSION THREAD

Ask your questions here. Or, answer questions of others here. Either way, you can learn.
DISCUSSION and HINTS
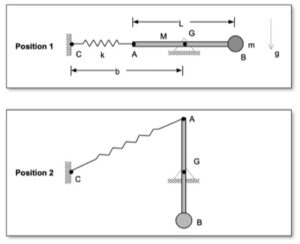
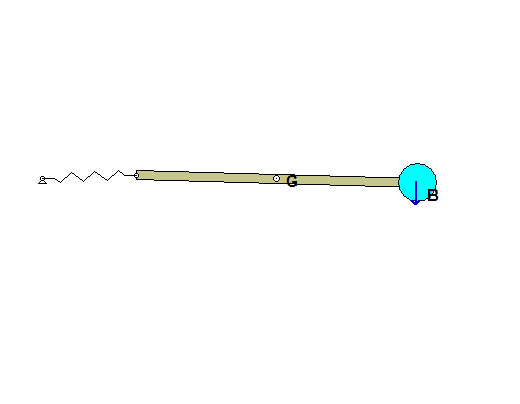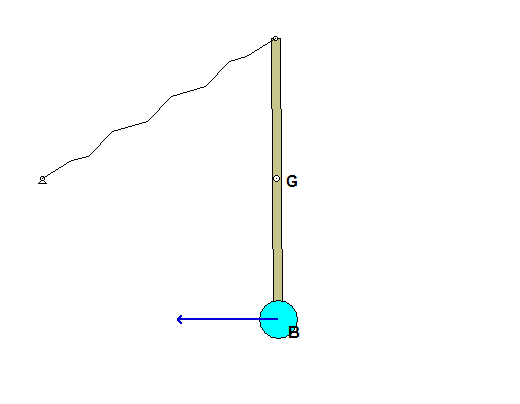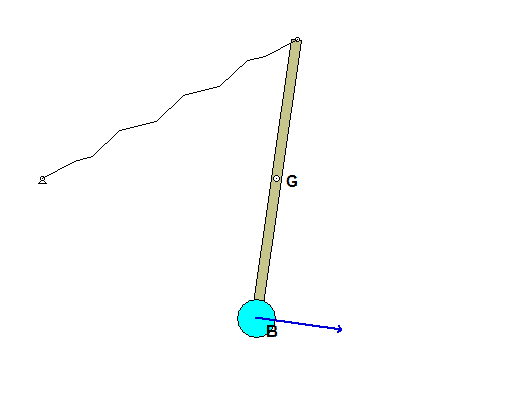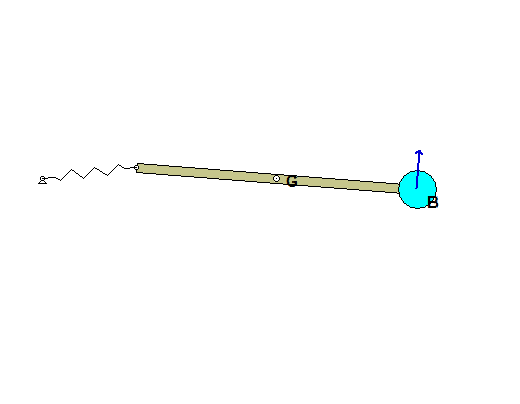
As the bar falls from its initial position, block B is moving downward. Once the bar has moved to a position where bar OA is aligned with section AC of the cable, the direction of motion for B is reversed and B begins moving upward. As can be seen in the animations, at some points in the subsequent motion, the cable goes in and out of being slack. What causes the cable to go slack?

Recall the following four-step plan outline in the lecture book and discussed in lecture:
Step 1: FBDs
Draw a single free body diagram (FBD) of the system made up of bar OA and block B.
Step 2: Kinetics (work/energy)
- Looking at your FBD above, which forces, if any, do work that is not a part of the potential energy of the system? If there are none, then energy is conserved.
- Write down the individual kinetic energy expressions for bar OA and block B, and add together for the total KE of the system.
- Define your gravitational datum line. Write down the individual potential energy expressions for the bar OA and block B, and add together for the total PE of the system.
Step 3: Kinematics
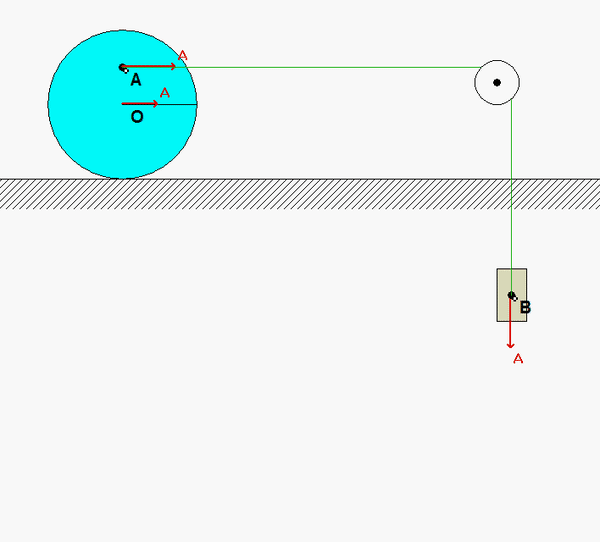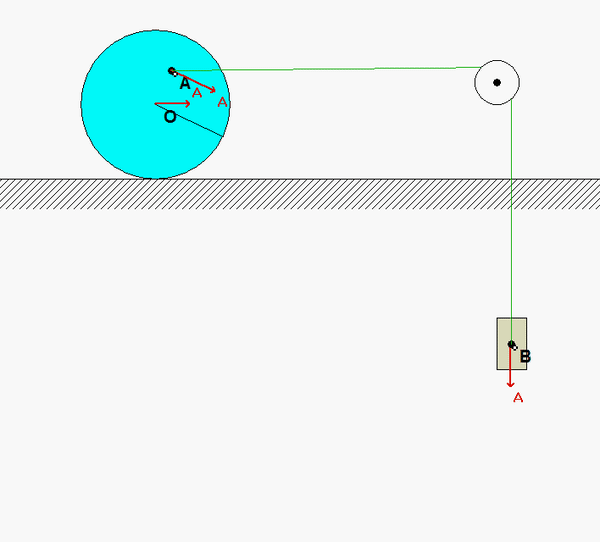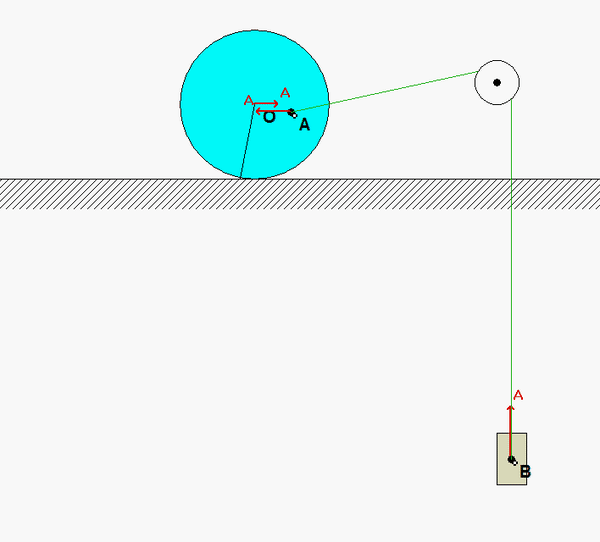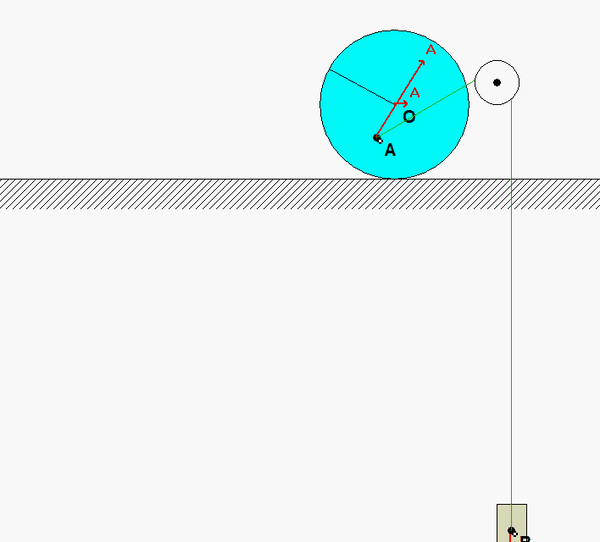
Relate the speeds of A and B, and relate the speed of A to the angular speed of bar OA. It is recommended that you use some polar coordinates with the origin at C. See the notes that follow. Note that since the cable does not stretch, the value of R_dot is the same as the speed of B.

A freeze-frame of the above animation is shown below for the position where OA is horizontal. Note that the speed of A is larger than the speed of B, as is indicated in the kinematic analysis above.

Step 4: Solve
From your equations in Steps 2 and 3, solve for the velocity of block B at position 2.