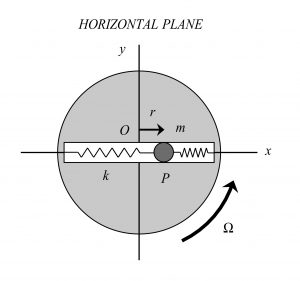
Particle P is able to slide within a smooth slot cut into a disk. The disk is rotating at a constant rate, Ω. We desire to understand the reaction force of the disk slot on the particle as the particle moves back and forth within the slot.

For the analysis, we will use a moving reference frame attached to the rotating disk. Using this in Newton's 2nd law, we determine the contact force F acting on the particle. It is seen that the contact force F is directly dependent on the Coriolis component of acceleration. Note that the sign on F changes as the sign on x_dot changes; that is, when the particle is moving from A to B (x_dot > 0) the contact force has a different sign from what it has when the particle is from B to A (x_dot < 0).
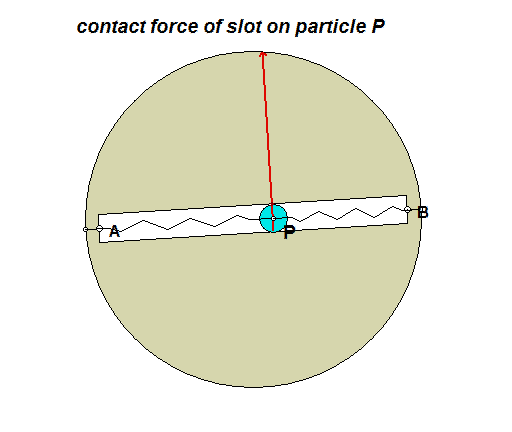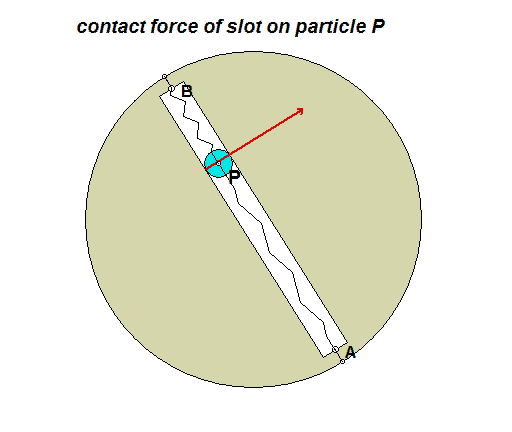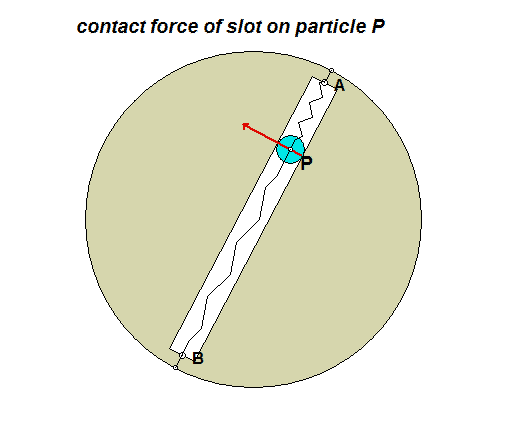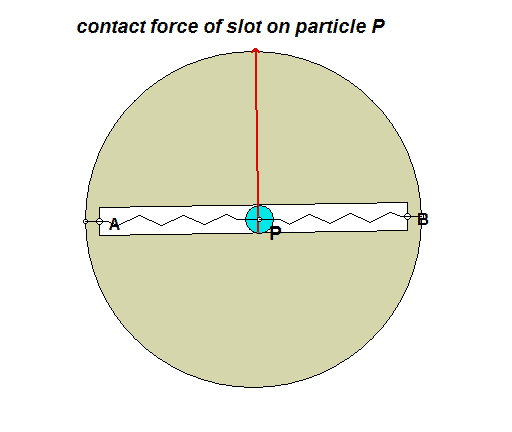
The simulation results above shows how the sign of the contact force (and hence the direction of the force) changes when the direction of motion of the particle within the slot changes. Note also that since x_dot appears to be the largest when the particle is at the center of rotation of the disk, this contact force has its maximum magnitude at that position.